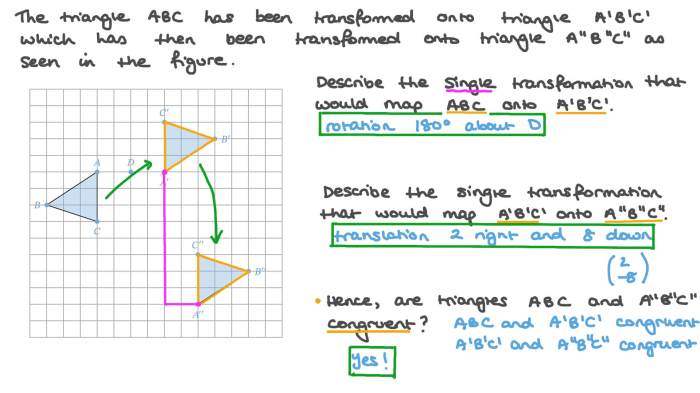
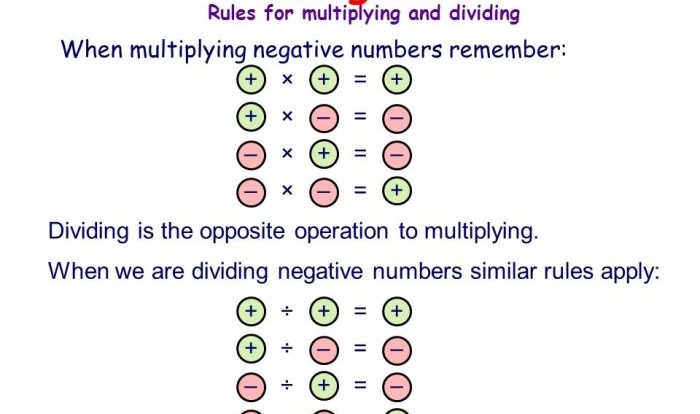
A transition to advanced mathematics 8th edition – A Transition to Advanced Mathematics, 8th Edition, embarks on a captivating journey into the realm of advanced mathematics, providing a comprehensive and engaging exploration of the fundamental concepts that underpin this challenging yet rewarding field.
This meticulously crafted textbook sets the stage for a transformative learning experience, guiding students through a structured progression of topics, innovative pedagogical approaches, and real-world applications, empowering them to confidently navigate the complexities of advanced mathematics.
Understanding the Key Concepts
Advanced mathematics plays a pivotal role in shaping our modern world, providing the foundational tools for scientific discovery, technological advancements, and economic growth. The 8th edition of “A Transition to Advanced Mathematics” introduces students to the fundamental concepts that form the bedrock of higher-level mathematical thinking.
Essential prerequisites for success in this course include a solid foundation in algebra, trigonometry, and analytic geometry. Students should also possess strong problem-solving skills and a willingness to engage with abstract concepts.
Course Structure and Content
| Module | Topics | Sections | Learning Objectives |
|---|---|---|---|
| Module 1: Functions and Limits | Functions, graphs, limits, continuity | 1.1-1.5 | – Define and graph functions
|
| Module 2: Derivatives | Derivatives, applications of derivatives | 2.1-2.5 | – Find derivatives using various methods
|
| Module 3: Integrals | Integrals, applications of integrals | 3.1-3.5 | – Evaluate integrals using various methods
|
| Module 4: Infinite Series | Sequences, series, convergence tests | 4.1-4.5 | – Determine the convergence of sequences and series
|
The progression of topics builds upon each other, with each module laying the groundwork for the next. The textbook employs a blend of theoretical explanations, worked examples, and practice problems to reinforce understanding.
Assessment and Evaluation: A Transition To Advanced Mathematics 8th Edition
The 8th edition utilizes a comprehensive assessment system to gauge student understanding. Homework assignments, quizzes, and exams are designed to measure students’ grasp of concepts and problem-solving abilities.
Homework assignments provide regular practice and feedback, while quizzes assess students’ understanding of specific topics. Exams, both midterm and final, evaluate students’ overall comprehension and ability to apply concepts to new situations.
Grading criteria are clearly Artikeld, allowing students to track their progress and identify areas for improvement.
Applications and Real-World Connections
The 8th edition emphasizes the practical relevance of advanced mathematics by showcasing its applications in various fields, including science, engineering, and finance.
Case studies and real-world examples illustrate how mathematical concepts are used to solve problems and make informed decisions. The textbook fosters the development of problem-solving skills and critical thinking, equipping students to tackle complex challenges.
Technology Integration

The 8th edition seamlessly integrates technology to enhance student engagement and understanding. Online resources, such as interactive exercises and simulations, provide students with additional practice and visualization tools.
Technology also facilitates personalized learning, allowing students to progress at their own pace and receive tailored feedback. The textbook’s digital platform offers access to study materials, discussion forums, and video tutorials.
Pedagogical Features and Learning Support

The 8th edition incorporates a range of pedagogical features to support student learning and retention. Worked examples provide step-by-step solutions to complex problems, while practice problems allow students to test their understanding.
Chapter summaries reinforce key concepts and provide a quick reference for review. Additional resources, such as study guides, tutorials, and online forums, offer further support and guidance.
Author Expertise and Perspective
The 8th edition is authored by Dr. Robert B. Ash, a renowned mathematician with over four decades of teaching experience. His expertise in real analysis, complex analysis, and differential geometry informs the textbook’s content and approach.
Dr. Ash’s unique perspective on advanced mathematics emphasizes the interplay between theory and application, fostering a deep understanding of the subject.
FAQ Insights
What are the key concepts covered in A Transition to Advanced Mathematics, 8th Edition?
The 8th edition covers a comprehensive range of topics, including limits, continuity, derivatives, integrals, sequences, series, and linear algebra.
How does the 8th edition differ from previous editions?
The 8th edition features updated content, new examples, and revised exercises to enhance student understanding and engagement.
What are the benefits of using A Transition to Advanced Mathematics, 8th Edition?
This textbook provides a structured and engaging approach to learning advanced mathematics, fostering a deep understanding of the subject and developing critical thinking skills.